How To Reconstruct Linked, Convergent and Serial Arguments with Argunet

by

Linked, convergent and serial argumentation are basic notions of argument structure in Critical Thinking and Informal Logic. This post describes how these argument patterns translate into Argunet argument maps.
LINKEDCONVERGENTSERIAL_TEASER

In a helpful review article Franciska Snoek Henkemans reminds us that in

most approaches, at least three types of argument structure are distinguished: (1) serial reasoning (or subordinate argumentation), (2) linked reasoning (or coordinate argumentation), and (3) convergent reasoning (or multiple argumentation). (p. 447)

She explains:

Reasoning is serial if one of the reasons supports the other. If reasoning is linked, each of the reasons given are directly related to the standpoint, and the reasons work together as a unit. When each reason separately supports the standpoint (to some degree), the reasoning is convergent. A complex argument can combine all of these types of argument structure. (p. 447)

Reconstructing linked argumentation

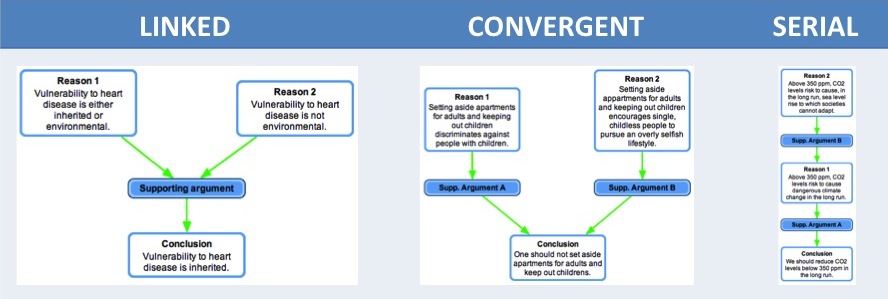
Here’s an example of a linked reasoning (from T. Govier’s A Practical Study of Argument, pp. 37-38):

linked_arg-1

The two reasons jointly support the conclusion, i.e., they are part of one and the same argument that justifies the conclusion. This argument is:

  1. Vulnerability to heart disease is not environmental.
  2. Vulnerability to heart disease is either inherited or environmental.
  3. Thus: Vulnerability to heart disease is inherited.

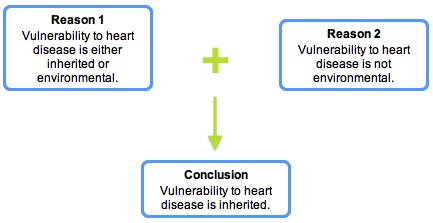
And the Argunet argument map which represents the linked reasoning looks as follows:

linked_arg-2

Reconstructing convergent argumentation

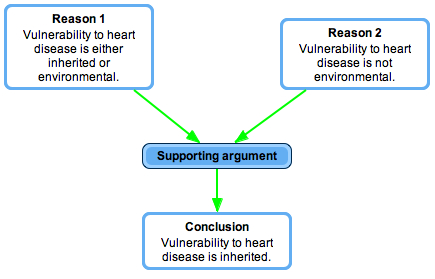
Here’s an example of a convergent reasoning (again from T. Govier’s A Practical Study of Argument, pp. 38-39):

convergent_arg-1

The two reasons support the conclusion independently of each other. That is, they give rise to two independent arguments, which might be reconstructed as follows.

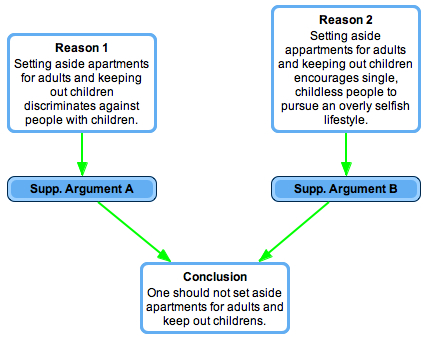
Supporting Argument A:

  1. Setting aside apartments for adults and keeping out children discriminates against people with children.
  2. Whatever discriminates against people with children should not be done. [Implicit premiss]
  3. Thus: One should not set aside apartments for adults and keep out children.

Supporting Argument B:

  1. Setting aside apartments for adults and keeping out children encourages single, childless people to pursue an overly selfish lifestyle.
  2. Whatever encourages single, childless people to pursue an overly selfish lifestyle should not be done.
  3. Thus: One should not set aside apartments for adults and keep out children.

The Argunet argument map of the convergent argument reads:

convergent_arg-2

Reconstructing serial argumentation

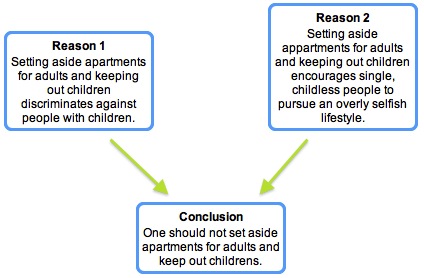
Finally, consider this case of serial reasoning:

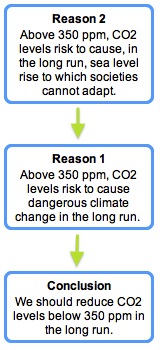
serial_arg-1

Reason 2 supposedly supports reason 1, which backs the conclusion. The serial reasoning can be reconstructed as a chain of two arguments, such that the conclusion of Argument B is a premiss of Argument A.

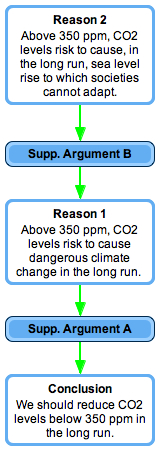
Supporting Argument B:

  1. Above 350 ppm, CO2 levels risk to cause, in the long run, sea level rise to which societies cannot adapt.
  2. Whatever risks to cause, in the long run, sea level rise to which societies cannot adapt, risks to cause dangerous climate change in the long run. [Implicit premiss]
  3. Thus: Above 350 ppm, CO2 levels risk to cause dangerous climate change in the long run. [Premiss 1 of Argument A]

Supporting Argument A:

  1. Above 350 ppm, CO2 levels risk to cause dangerous climate change in the long run.
  2. If, above a level of X, CO2 levels risk to cause dangerous climate change in the long run, then we should reduce CO2 levels below X in the long run. [Implicit premiss]
  3. Thus: We should reduce CO2 levels below 350 ppm in the long run.

And this is the corresponding argument map:

serial_arg-2

So, linked, convergent and serial argumentation can be reconstructed in Argunet.

Comments are closed.